(Study Materials) Numerical Ability/Quantitative Aptitude : Probability
(Study Materials) Numerical Ability/Quantitative Aptitude : Probability
Probability
Probability is used to indicate a possibility of an event to occur. It is often used synonymously with chance.
- In any experiment if the result of an experiment is unique or certain, then the experiment is said to be deterministic in nature.
- If the result of the experiment is not unique and can be one of the several possible outcomes then the experiment is said to be probabilistic in nature.
Various Terms Used in Defining Probability
(i) Random Experiment: Whenever an experiment is conducted any number of
times under identical conditions and if the result is not certain and is any
one of the several possible outcomes, the experiment is called a trial or a
random experiment, the outcomes are known as events.
eg, When a die is thrown is a trial, getting a number 1 or 2 or 3 or 4 or 5
or 6 is an event.
(ii) Equally Likely Events: Events are said to be
equally likely when there is no reason to expect any one of them rather than
any one of the others.
eg, When a die is thrown any number 1 or 2 or 3 or 4 or 5 or 6 may occur. In
this trial, the six events are equally likely.
(iii) Exhaustive Events: All the possible events in any trial are known as exhaustive events. eg, When a die is thrown, there are six exhaustive events.
(iv) Mutually Exclusive Events: If the occurrence of any one of the events in a trial prevents the occurrence of any one of the others, then the events are said to be mutually exclusive events. eg, When a die is thrown the event of getting faces numbered 1 to 6 are mutually exclusive.
Classical Definition of Probability
If in a random experiment, there are n mutually exclusive and equally likely elementary events in which n elementary events are favourable to a particular event E, then the probability of the event E is defined as P (E)
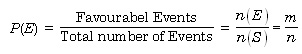
• If the probability of occurrence of an event E is P(E) and the probability of non-occurrence is P, then,
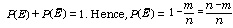the sum of the probabilities of success and failure is 1. Also, 0
≤ P(E) ≤
1 and 0 ≤ P ≤
1.
• If P(E) = 1, the event E is called a certain event and if P(E) = 0, the event E is called an impossible event.
• If E is an event, then the odds in favour of E are defined as P(E) : P(E) and the odds against E are defined
as
P(E): P. Hence, the odds in favour of E are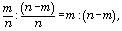
the odds against E are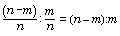
Addition Theorem on Probability
Independent and Dependent Events
- Simple Event : An event which cannot be further split is called a simple event. The set of all simple events in a trial is called a sample space.
- Compound Event : When two or more events occur in relation with each other, they are called compound events.
- Conditional Event: If El and E2 are events of a sample space S and if E2 occurs after the occurrence of El, then the event of occurrence of E2 after the event El is called conditional event of E2 given El. It is denoted by E2/El.
‘Smart’ Facts
- When a die is rolled six events occur. They are {1, 2, 3, 4, 5 and 6}
- When two dice are rolled 36 events occur. They are [(1,1), (1,2), (1,3), (1,4), (1,5), (1,6), (2,1), (2,2), (2,3), (2,4), (2,5), (2,6), (3,1), (3,2), (3,3), (3,4), (3,5), (3,6), (4,1), (4,2), (4,3), (4,4), (4,5), (4,6), (5,1), (5,2), (5,3), (5,4), (5,5), (5,6), (6,1), (6,2), (6,3), (6,4), (6,5), (6,6)]
- When a coin is tossed 2 events occur. They are {H, T}
- When two coins are tossed 4 events occur. They are {HH, HT, TH, T T}
- When three coins are tossed 8 events occur. They are {HHH HHT, HTH, HT T, T HH, THT, T TH, T T T}
- In a pack of 52 cards there are 26 red cards and 26 black cards. The 26 red cards are divided into 13 heart cards and 13 diamond cards. The 26 black cards are divided into 13 club cards and 13 spade card. Each of the colours, hearts, diamonds, clubs and spades is called a suit. In a suit, we have 13 cards (ie, A, K, Q, J, 10, 9, 8, 7, 6, 5, 4, 3 and 2)
